This question is about arrangements of all eight letters in the word IMMUNITY.

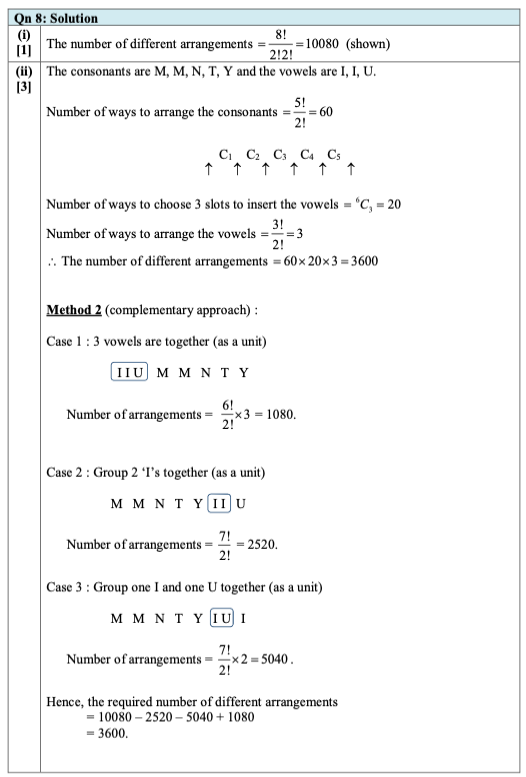
(i) Show that the number of different arrangements of the eight letters that can be made is 10080 .

[1]

(ii) Find the number of different arrangements that can be made with no two vowels next to each other.

[3]

One of the 10080 arrangements in part (i) is randomly chosen.

Let (A) denote the event that the two I’s are next to each other and let (B) denote the event that the two M’s are next to each other.

(iii) Determine, with a reason, whether (A) and (B) are

(a) mutually exclusive,

[1]

(b) independent.

[3]

(iv) Find the probability that the chosen arrangement contains no two adjacent letters that are the same.

[4]