$\left[\right.$ It is given that the volume of a sphere of radius $R$ is $\frac{4}{3} \pi R^3$.
Craft drinks have been gaining popularity in the beverage industry in recent years. These drinks are usually freshly made and served cold, with much attention given to the ingredients that make up the drinks and the entire process of preparation.
Ice is a very important ingredient in the making of a craft drink as it affects two crucial components: the temperature and the dilution of the drink. Hence, great emphasis is placed on the shapes of the ice, as different shapes will offer different surface areas and thus have a direct impact on the taste of the drink.
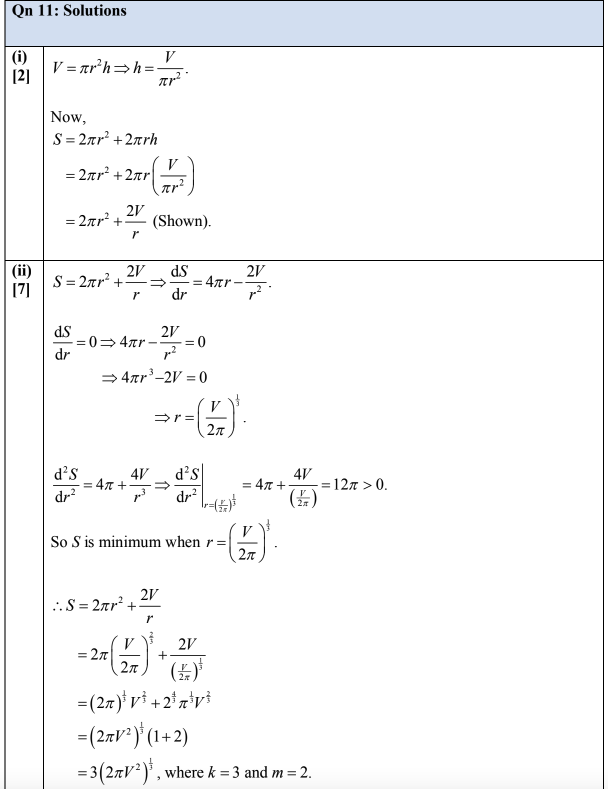
(i) A piece of cylindrical shaped ice has radius $r$, height $h$ and a fixed volume $V$. Show that its surface area, $S$, is given by $2 \pi r^2+\frac{2 V}{r}$.
[2]
ii) Use differentiation to find, in terms of $V$, the minimum value of $S$, proving that it is a minimum. You are to give your answer in the form $k\left(m \pi V^m\right)^{\frac{1}{k}}$, where $k$ and $m$ are positive integers to be found. Find also the ratio $r: h$ that gives this minimum value of $S$.
[7]
(iii) For the minimum value of $S$ found in part (ii), show that the volume of the largest spherical shaped ice that can be carved out is $\frac{m}{k} V$, where $k$ and $m$ are the same integers found in part (ii).
[2]
(iv) State, giving a reason, whether the manufacturer should proceed to carve out spherical shaped ice from the existing cylindrical shaped ice produced.
[1]
(ii) \(r: h=1: 2\)
(iv)
No, the manufacturer should not proceed as the spherical shaped ice has volume at least \(\frac{2}{3} V\) and so \(\frac{1}{3}\) of the volume of the cylindrical shaped ice will go to waste which is quite a lot.
OR
Yes, the manufacturer should proceed even though the spherical shaped ice has volume at least \(\frac{2}{3} V\) as the \(\frac{1}{3} V\) of crushed ice that is leftover during carving can be used for other drinks which require crushed ice.
When $r=\left(\frac{V}{2 \pi}\right)^{\frac{\dagger}{3}}, \frac{r}{h}=\frac{r}{\left(\frac{V}{\pi r^2}\right)}=\frac{\pi r^3}{V}=\frac{\pi\left(\frac{V}{2 \pi}\right)}{V}=\frac{1}{2}$.
