Question
Answer Key
Worked Solution
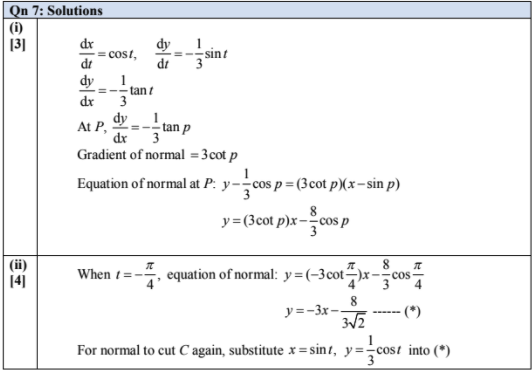
RI/2021/JC2/Prelim/P1/Q07
A curve $C$ has parametric equations
$$
x=\sin t, \quad y=\frac{1}{3} \cos t, \text { for }-\pi \leq t \leq \frac{\pi}{4} .
$$
(i) Find the equation of the normal to $C$ at the point $P$ with parameter $t=p$.
[3]
(ii) The normal to $C$ at the point when $t=-\frac{\pi}{4}$ cuts the curve again at point $A$. Find the coordinates of point $A$, correct to 2 decimal places.
[4]
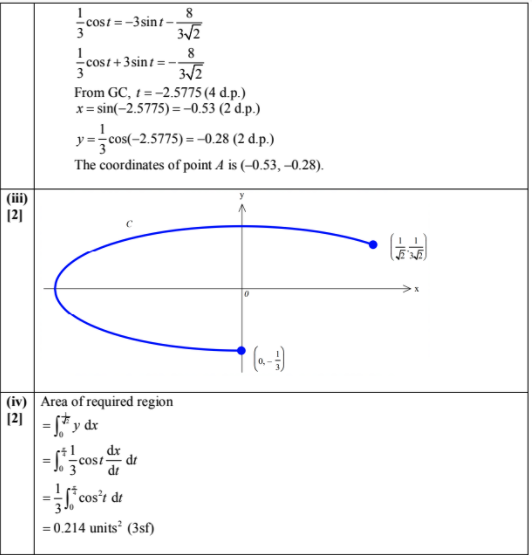
(iii) Sketch the graph of $C$, giving the coordinates of the end points in exact form.
[2]
(iv) Find the area of the region bounded by $C$, the $x$-axis and the lines $x=0$ and $x=\frac{1}{\sqrt{2}}$.
[2]
(i) \(y=(3 \cot p) x-\frac{8}{3} \cos p\)
(ii) \((-0.53,-0.28)\)
(iii)

(iv) \(0.214\) units \(^{2}(3 sf )\)