9758/2020/P2/Q06

In this question you should assume that $T, W$ and $D$ follow independent normal distributions.

James leaves home to go to work at $T$ minutes past 8 am each day, where $T$ follows the distribution $\mathrm{N}\left(5,1.2^{2}\right)$

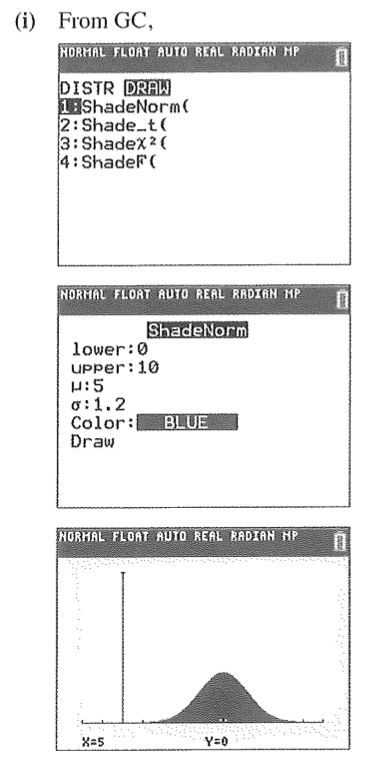
(i) Sketch this distribution for the period from $8 \mathrm{am}$ to $8.10 \mathrm{am}$.

[2]

(ii) Find the probability that, on a randomly chosen day, James leaves for work later than $8.06$ am.

[1]

When the weather is fine, James walks to work. The time, $W$ minutes, he takes to walk to work follows the distribution $\mathrm{N}\left(21,3^{2}\right)$. James is supposed to start work at $8.30 \mathrm{am}$.

(iii) Find the probability that, on a randomly chosen day when James walks, he is late for work.

[2]

When the weather is not fine, James drives to work. He still leaves at $T$ minutes past 8 am each day; the time, $D$ minutes, he takes to drive to work follows the distribution $\mathrm{N}\left(19,6^{2}\right)$.

On average, the weather is fine on $70 \%$ of mornings.

(iv) One day, James is late for work. Find the probability that the weather is fine that day.

[5]

(ii)

(iv) $\mathrm{E}(T+D)=\mathrm{E}(T)+\mathrm{E}(D)$
$=5+19$
$=24$
$\operatorname{Var}(T+D)=\operatorname{Var}(T)+\operatorname{Var}(D)$
$=1.2^2+6^2$
$=37.44$
$T+D \sim \mathrm{N}(24,37.44)$

(ii) $\mathrm{P}(T>6)=0.202$ (to 3 s.f.)

[A1]

(iii) $\mathrm{E}(T+W)=\mathrm{E}(T)+\mathrm{E}(W)$
$=5+21$
$=26$
$\operatorname{Var}(T+W)=\operatorname{Var}(T)+\operatorname{Var}(W)$
$=1.2^2+3^2$
$T+W \sim \mathrm{N}(26,10.44)$

[B1]

$\mathrm{P}(T+W>30)$
$=0.10786$ (to 5 s.f.)
$=0.108$ (to 3 s.f. $)$

[A 1]

$\mathrm{P}$ (weather is fine | James is late)
$=\frac{\mathrm{P} \text { (weather is fine and James is late })}{\mathrm{P} \text { (James is late })}$
$=\frac{0.7 \times \mathrm{P}(T+W>30)}{0.7 \times \mathrm{P}(T+W>30)+0.3 \times \mathrm{P}(T+D>30)}$

[B1]

$=\frac{0.7 \times 0.10786}{0.7 \times 0.10786+0.3 \times 0.16340}$

[B1]

$=0.606$ (to 3 s.f.)

[A1]